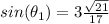Answer:
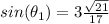
Explanation:
The complete question is
The angle θ1 is located in Quadrant 1, and cos (θ1)=10/17.
What is the value of sin(θ1)?
we know that
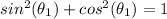 ---> trigonometric identity
---> trigonometric identity
we have
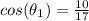
The angle
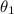 is located in Quadrant I, that means the sine of angle
is located in Quadrant I, that means the sine of angle
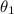 is positive
is positive
substitute the given value in the trigonometric identity
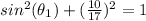
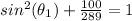
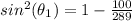
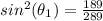
take square root both sides
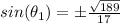
Remember that the sine is positive (Quadrant I)
so
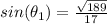
Simplify