Answer:

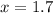
Hopefully the answers are accepted to the nearest tenths.
Explanation:
Since the left hand side was already graphed, we just need to graph the right hand side which is
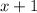 .
.
We are going to still let
 represent the outputs of
represent the outputs of
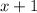 .
.
So we want to graph the equation
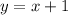 on the same graph as the parabola, the almost U shaped graph.
on the same graph as the parabola, the almost U shaped graph.
You could make a table to plot the equation.
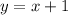
 |
|
 | ordered pair to graph
| ordered pair to graph
-------------------------------------------------------------------------------------------------------
-2 -2+1=-1 (-2,-1)
-1 -1+1=0 (-1,0)
0 0+1=1 (0,1)
1 1+1=2 (1,2)
2 2+1=3 (2,3)
The purple line I made is the line formed from these points which was generated from the equation
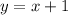 .
.
Now where the blue curve touches the purple line is our solutions. I put those in orange.
The solutions are approximately,
 or
or
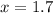 .
.
Hopefully, it accepts solutions to the nearest tenths.
We are trying to approximate where those orange dots are on the number line. Please see attachment 2.