Answer:
(-6,16), also can be written as x=-6, y=16.
Explanation:
By 'sub method' I assume you mean substitution of one solved equation into another. Let's begin by solving x+y=10 for y.
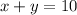
-x on both sides

Now we have an equation that tells us y=10-x. We can now replace 'y' in the other equation with this new definition of y, that being 10-x.
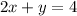
Substitute 10-x for y
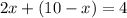
When can remove the parentheses as they are unnecessary.
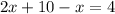
Now we solve for x. We can first simplify, by combining all like terms on each side, those being 2x and -x on the left side. 2x+-x=x.
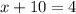
Now we remove 10 from both sides, to isolate x.

We can again simplify, 4-10=-6

Now we have the x coordinate of the point that solves our set of equations. We can plug this number back as an x value of either equation to get the y value for the solution. In this example I will use x+y=10, as it is a simpler equation.
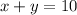
Substitute x for -6.
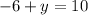
Isolate y by adding 6 to both sides, cancelling out the -6 on the left side.
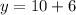
Simplify 10+6=16.
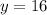
Now we have an x value, -6, and a y value, 16. These are the x and y values of our solution, therefore the solution is (-6,16).
This is the solution using the sub method. There are other ways to solve this question (although the answer, if done correctly, will always be the same), including plotting both lines on a graph, as shown in the attached image.