Answer:

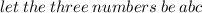
as they are in continued proportion

given that mean proportional is 12
: b=12
so
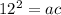
144 = ac
c=144\a
the sum of the two numbers
a+c=26
a+144\a=26
step 2: simplify using factorization
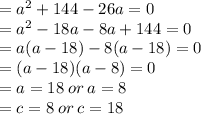
a=18 or a=8
c=8 or c=18
hence the numbers are 8,12,18 or 18,12,8
Was this answer helpful