Answer:
x = 1, y = 60 or (1, 60)
Explanation:
Given the quadratic function, f(x) = -60x² + 120x, which models a reverse bungee jump. If we graph this given quadratic function, then it will show a downward-facing parabola whose vertex is the maximum point on the graph.
Solution:
We can determine the maximum height of the given quadratic function by using the formula to find the x-coordinate of the vertex:
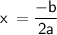
From the given quadratic function: f(x) = -60x² + 120x, where a = -60, b = 120, and c = 0:
Substitute the given values for a and b into the formula for finding the x-coordinate value of the vertex:
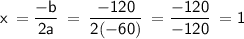
Now that we have the value for the x-coordinate of the vertex, x = 1, substitute this value into the given quadratic function to find its corresponding y-coordinate:
f(x) = -60x² + 120x
f( 1 ) = -60( 1 )² + 120( 1 )
f( 1 ) = -60 + 120
f( 1 ) = 60
Thus, the y-coordinate of the vertex is f(x) = 60.
Final Answer:
Therefore, the maximum height of the reverse bungee jump is x = 1, y = 60 or (1, 60).