Question

A circle with radius of 3 cm sits inside a circle with radius of 11 cm. What is the area of the shaded region?

Answer 1

The area of shaded region is 351.68 cm².

Explanation:

`\sf{\underline{\underline{Given :}}}`

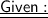
• ➝ Radius of small circle = 3 cm
• ➝ Radius of big circle = 11 cm

`\sf{\underline{\underline{To \: Find:}}}`

• ➝ Area of small circle
• ➝ Area of big circle
• ➝ Area of shaded region

`\sf{\underline{\underline{Using\: Formula :}}}`

• ➝ Area of small circle = πr²
• ➝ Area of big circle = πR²
• ➝ Area of shaded region = Area of small circle - Area of big circle

`\sf{\underline{\underline{Solution :}}}`

Finding the area of small circle by substituting the values in the formula :

• 
  `\red\implies` Area of small circle = πr²
• 
  `\red\implies` 3.14 × 3²
• 
  `\red\implies` 3.14 × 3 × 3
• 
  `\red\implies` 28.26cm²

Hence, the area of small circle is 28.26cm².

`\rule{200}2`

Finding the area of big circle by substituting the values in the formula :

• 
  `\pink\implies` Area of big circle = πR²
• 
  `\pink\implies` 3.14 × 11²
• 
  `\pink\implies` 3.14 × 11 × 11
• 
  `\pink\implies` 379.94cm²

Hence, the area big circle is 379.94cm².

`\rule{200}2`

Finding the area of shaded region by substituting the values in the formula :

• 
  `\orange\implies` Area of shaded region = Area of bigger circle - Area of smaller circle
• 
  `\orange\implies` 379.94 - 28.26 cm²
• 
  `\orange\implies` 351.68 cm²

Hence, the area of shaded region is 351.68 cm².

`\underline{\rule{220pt}{3.5pt}}`