Answer:
A. Slope is -12 feet per second
B. Yes, it is constant.
Skills needed: Linear Equations, Substitution and Division
Explanation:
1) Solving Part A (we need to find the slope):
- The slope is
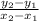 -->
-->
 ,
,
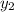 ,
,
 , and
, and
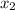 are all values from the table. We know that the left column is the x-values, and the right column is the y-values as that is the conventional way of depicting them.
are all values from the table. We know that the left column is the x-values, and the right column is the y-values as that is the conventional way of depicting them.
2) Using the y-values of 1150 and 1090, and their corresponding x-values (5 and 10 respectively), we can get the slope:
-
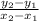 ==>
==>
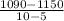 ==>
==>
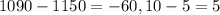 ==>
==>
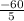
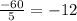 , so the slope is -12.
, so the slope is -12.
==================================================================
1) Part B (analysis)
We can see that no matter what 2 y-values and their corresponding x values we use, the slope always is the same. This means that the rate of change is constant.