Answer:
It is an identity, proved below.
Explanation:
I assume you want to prove the identity. There are several ways to prove the identity but here I will prove using one of method.
First, we have to know what cot and cosec are. They both are the reciprocal of sin (cosec) and tan (cot).
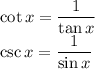
csc is mostly written which is cosec, first we have to write in 1/tan and 1/sin form.
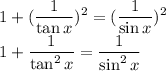
Another identity is:
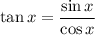
Therefore:
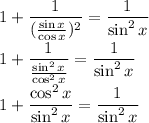
Now this is easier to prove because of same denominator, next step is to multiply 1 by sin^2x with denominator and numerator.
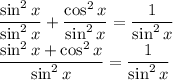
Another identity:
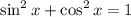
Therefore:

Hence proved, this is proof by using identity helping to find the specific identity.