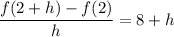Answer:
8+h
Explanation:
Simply substitute x = 2+h and x = 2 in.
![\displaystyle \large{(f(2+h)-f(2))/(h)=((2+h)^(2) +4(2+h)+5-[(2)^2+4(2)+5])/(h)}\\\displaystyle \large{(f(2+h)-f(2))/(h)=(4+4h+h^2 +8+4h+5-17)/(h)}\\\displaystyle \large{(f(2+h)-f(2))/(h)=(h^2 +8h)/(h)=h+8}](https://img.qammunity.org/2022/formulas/mathematics/college/j89mc1q3mfmurned5atg5mkj9dqxr9ltpj.png)
Therefore, the answer is h+8 or 8+h.
Another way is to differentiate the function with respect to x. This equation or expression is rate of changes from 2 to 2+h with h being any numbers.
The definition of derivative is:
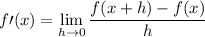
Notice how both f(2+h)-f(2) over h and the definition of derivative look same except the derivative has limit of h approaching to 0, making h not having any values by default.
Since f(2+h)-f(2) over h does not have limit, we have to add +h when differentiating the function.
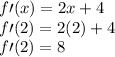
Since f’(2) is 8 but because f(2+h)-f(2)/h does not have limit of h, therefore we add +h.