Answer:
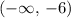 and
and
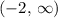 .
.
Explanation:
A continuous function is increasing if and only if its first derivative is positive.
Differentiate
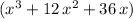 with respect to
with respect to
 to find the first derivative of this function.
to find the first derivative of this function.
![\begin{aligned}& (d)/(d x) \left[x^(3) + 12\, x^(2) + 36\, x\right] \\ =\; & 3\, x^(2) + 24\, x + 36 \\ =\; & 3\, (x^(2) + 8\, x + 12)\end{aligned}](https://img.qammunity.org/2022/formulas/mathematics/high-school/bifets839mmmvfl1lb3k2m1jzsy3ol4hep.png) .
.
In other words, the given function is increasing if and only if
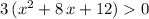 .
.
Notice that
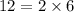 while
while
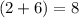 . Thus, the quadratic expression
. Thus, the quadratic expression
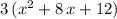 may be rewritten as:
may be rewritten as:
 .
.
Thus, the requirement that
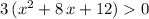 is equivalent to
is equivalent to
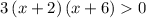 , which is true if and only if:
, which is true if and only if:
Equivalently,
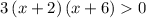 is true if and only if:
is true if and only if:
The first requirement simplifies to
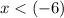 and corresponds to the interval
and corresponds to the interval
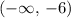 .
.
The second requirement simplifies to
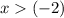 , which corresponds to the interval
, which corresponds to the interval
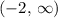 .
.
Thus, the given function is increasing on the interval
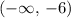 and
and
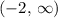 .
.