Question

Find the distance between each pair of points (0,3) (5,-3)

Answer 1

`√(61)`

Explanation:

Calculate the distance d using the distance formula

d =
`\sqrt{(x_(2)-x_(1))^2+(y_(2)-y_(1))^2 }`

with (x₁, y₁ ) = (0, 3 ) and (x₂, y₂ ) = (5, - 3 )

d =
`√((5-0)^2+(-3-3)^2)`

=
`√(5^2+(-6)^2)`

=
`√(25+36)`

=
`√(61)`

Answer 2

`\displaystyle\mathsf{Distance(d)\:=\:√(61)\:\:or\:\:7.8102}`

Explanation:

Given the two points, (0, 3) and (5, -3), we could use the distance formula to determine how far apart are these two points from each other.

Let (x₁, y₁) = (0, 3)

(x₂, y₂) = (5, -3)

Substitute these values into the following distance formula:

`\displaystyle\mathsf{Distance(d)\:=\:√((x_2\:-\:x_1)^2 \:+\:(y_2\:-\:y_1)^2) }`

`\displaystyle\mathsf{Distance(d)\:=\:√((5\:-\:0)^2 \:+\:(-3\:-\:3)^2) }`

`\displaystyle\mathsf{Distance(d)\:=\:√((5)^2 \:+\:(-6)^2) }`

`\displaystyle\mathsf{Distance(d)\:=\:√(25 \:+\:36) }`

`\displaystyle\mathsf{Distance(d)\:=\:√(61)\:\:or\:\:7.8102}`

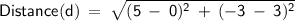
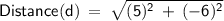
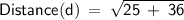
Therefore, the distance between the given pair of points is
`\displaystyle\mathsf{√(61)\:\:or\:\:7.8102}`.