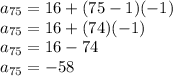Answer:
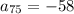
Explanation:
This is an arithmetic sequence:
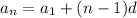
where d is the common difference and n is the index of any given term.
For the given sequence, the common difference is -1:
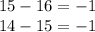
Knowing both the common difference and the first term, you can write the equation for this sequence:
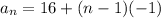
Then you can use that equation to find the 75th term: