Answer:
Between 3 and 4 hours
Explanation:
Let's turn the sentence into an equation, suppose that both trucks will be traveling at a constant rate, let x be the number of hours truck A has been traveling, and let y be the number of hours truck B has been traveling. Then we can set up the equation:
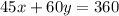
Both of these terms must add up to 360 since that will be the point where both trucks will meet. To solve this, we can use a graphing calculator:
(see picture)
Since both trucks left the warehouses at the same time, we need that:
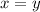
And the point in the graph that x=y is around 3.42 and 3.44, so we can conclude that both trucks must be traveling for 3-4 hours for them to meet on the road.
I hope this helps.