Answer: A) V=
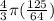 B) V=
B) V=
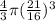 C)
C)
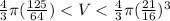
Step-by-step explanation:
A) A sphere with a diameter of 2 1/2 inches: This means the sphere is relatively smaller, and when you calculate its volume, it will be based on its size.
B) A sphere with a diameter of 2 5/8 inches: This refers to a slightly larger sphere, and its volume will be calculated based on its larger size.
When comparing the volumes of these two spheres, you can use an inequality. An inequality is a way of expressing that one value is larger or smaller than another without giving the exact numerical values. In this case, you're comparing the volumes, so you can say that the volume of the smaller sphere (A) is less than the volume of the larger sphere (B).
So, the inequality expressing the volume of a sphere between these two diameters would be:
Volume of A < Volume of B
This inequality indicates that the volume of the sphere with a diameter of 2 1/2 inches is smaller than the volume of the sphere with a diameter of 2 5/8 inches.