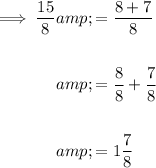Answer:

Explanation:
Subtract the used volume of punch from the starting volume of punch.
Convert the mixed number to an improper fraction:

Make the denominators of both fractions the same by multiplying the numerator and the denominator of 9/4 by 2:


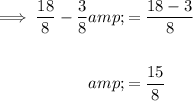
Convert back into a mixed number: