Question

PLEASE HELP...

Write an iterated integral of a continuous function f over the region. Use the order dy dx.
R is the triangular region with vertices ​(0​,0​), ​(0​,15​), and ​(5​,0​).

(I just need the double iterated integral no solving necessary)
THANK YOU!!

Answer 1

`\displaystyle \int_(0)^(5)\int_(0)^(-3x+15)f(x,y) \ dy dx`

========================================================

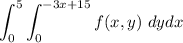
Step-by-step explanation:

We're tasked to find the values of a,b,c,d in the following

`\displaystyle \int_(a)^(b)\int_(c)^(d)f(x,y) \ dy dx`

The equation of the line through the points (0,15) and (5,0) is y = -3x+15

When picking a particular fixed x value, the y values span from y = 0 to y = -3x+15 which determines the bounds of integration for the inner integral.

So this means c = 0 and d = -3x+15

The values of a,b are much easier and they are a = 0 and b = 5 to represent the x coordinates of the left-most and right-most points.

-----------------

Since,

• a = 0
• b = 5
• c = 0
• d = -3x+15

we go from this

`\displaystyle \int_(a)^(b)\int_(c)^(d)f(x,y) \ dy dx`

to this

`\displaystyle \int_(0)^(5)\int_(0)^(-3x+15)f(x,y) \ dy dx`

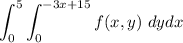
Without knowing what f(x,y) is, we cannot compute the integral. Luckily it seems like your teacher is only interested in setting up the integral rather than computing its numeric value.