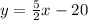Question

Write the equation of the line that is parallel to 5x - 2y =16 and passes through (10,5).

Group of answer choices

A. y =5/2x -30

B. y = 5/2x -20

C. y = -2/5x -15

D.y = -2/5x +30

Answer 1

B

Explanation:

Parallel lines have same slope. Parallel means to never intersect. So if something has to not intersect, they must have similar slopes but from a different starting position.

So Parallel Lines have similar slopes.

So using the given equation let manipulate this expression to find our a slope. This looks like a linear equation in the form of Ax+By=C (standard form). So let isolate this to y=mx+b because the latter is slope intercept form, which gives us our slope which is m.

`5x - 2y = 16`

We need to get it to look like

`y = mx + b`

So we need to isolate our y value.

In order to do this, we do basics of Algebra

`- 2y = 16 - 5x`

`y = (16 - 5x)/( - 2)`

`y = - 8 + (5)/(2) x`

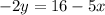
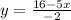
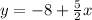
So our slope of the original line is 5/2. So our new Parallel lines must have this slope as well. Our new line also passes through 10,5 so since we have a point and a slope we use point slope form

`y - {y} _(1) = m( x - x _(1))`

where m is our slope and (x1,y1) is our given point.

Subsitue

`y - 5 = (5)/(2) (x - 10)`

`y - 5 = (5)/(2) x - 25`

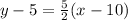
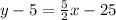
Add 5 to both sides

`y = (5)/(2) x - 20`