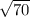Answer:
NM =
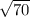
Explanation:
Δ NMO and Δ KML are similar ( by the AA postulate )
Then the ratios of corresponding sides are in proportion, that is
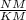 =
=
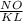 ( substitute values, noting KL = 2NM )
( substitute values, noting KL = 2NM )
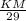 =
=
 ( cross- multiply )
( cross- multiply )
2 NM² = 140 ( divide both sides by 2 )
NM² = 70 ( take the square root of both sides )
NM =