Question

A ball is thrown from ground level at an angle of 0 and the initial velocity components are 7.5m/s and 13m/s. Ignoring air resistance, calculate each of:

1- The initial velocity is vi

2- The value of the angle at which the ball was thrown

3 - The horizontal range of the ball R​

Answer 1

I assume you mean an angle of θ, and not 0. I also assume the given components of the initial velocity are horizontal and vertical, respectively, so that

1. the initial velocity vector is

`\boxed{\vec v_i = (7.5\,\vec\imath + 13\,\vec\jmath) (\rm m)/(\rm s)}`

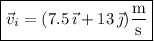
which means the ball is thrown with an initial speed of

√((7.5 m/s)² + (13 m/s)²) ≈ 15 m/s,

and

2. the angle made by
`\vec v_i` with the positive horizontal axis is θ such that

`\tan(\theta) = (13)/(7.5) \implies \theta \approx \arctan(1.7) \approx \boxed{60^\circ}`

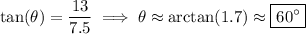
3. At time t, the ball attains a height y and horizontal range x according to

y = (13 m/s) t - g/2 t²

x = (7.5 m/s) t

where g = 9.8 m/s². When the ball reaches the ground (y = 0) for t > 0, we have

(13 m/s) t - g/2 t² = 0

13 m/s - g/2 t = 0

t = 2 (13 m/s)/g = (26 m/s)/g

Plugging this time into the x equation gives a horizontal range of

x = (7.5 m/s) (26 m/s)/g ≈ 20. m