Question

Can someone help me solve this question!
?

Answer 1

Key Concepts

• Algebraic equations from word problems
• Systems of equations

Solving the Question

We're given:

• There were 478 people in total.
• $1.75 per child
• $2.25 per adult
• Total revenue of $975
• We must find the number of children and adults who swam.

Set variables:

Let a represent the number of children who swam.

Let b represent the number of adults who swam.

Create equations

Because we've set two variables, we need two equations to be able to find the answers.

One of the equations will regard price while the other will regard the number of people, as those are the two things given in the question.

Number of people:

`a+b=478`

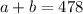
• The number of children + the number of adults = 478 in total

Price:

`1.75a+2.25b=975`

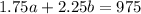
• $1.75 per child + $2.25 per child = $975 in total

Solve equations

• 
  `a+b=478`
• 
  `1.75a+2.25b=975`

We can solve this question using substitution, where we plug one equation into the other.

Isolate a in the first equation:

`a+b=478\\a=478-b`

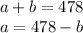
Plug the first equation into the second equation by replacing a:

`1.75a+2.25b=975\\1.75(478-b)+2.25b=975`

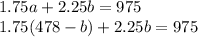
Solve for b:

`1.75(478-b)+2.25b=975`

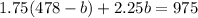
⇒ Open up the parentheses:

`836.5-1.75b+2.25b=975\\836.5-0.5b=975`

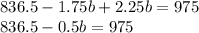
⇒ Subtract 836.5 from both sides to isolate b:

`-0.5b=138.5`

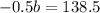
⇒ Divide both sides by -0.5:

`b=277`

• Therefore, there were 277 adults in total.

Solve for a:

`a+b=478`

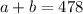
⇒ Replace b with 277:

`a+277=478\\a=201`

• Therefore, there were 201 children in total.

Answer

There were 201 children and 277 children at the pool that day.