Proof -
So, in the first part we'll verify by taking n = 1.
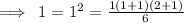
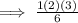
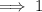
Therefore, it is true for the first part.
In the second part we will assume that,
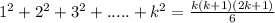
and we will prove that,
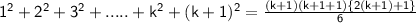
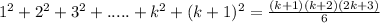

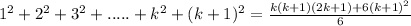
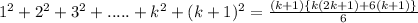
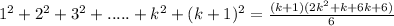
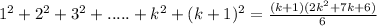

Henceforth, by using the principle of mathematical induction 1²+2² +3²+....+n² = n(n+1)(2n+1)/ 6 for all positive integers n.
_______________________________
Please scroll left - right to view the full solution.