Hello!
To solve, we can begin by using the kinematic equation:
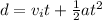
Where:
vi = initial velocity (m/s)
t = time (s)
a = acceleration (in this case, due to gravity. g = 9.8 m/s²)
Since the object falls from rest, the initial velocity is 0 m/s.
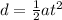
Plug in the given values:
