Question

If the parallel sides of a trapezium are 2 cm apart and their sum is 10 cm then find its area.

Answer 1

Given :

• The parallel sides of a trapezium are 2 cm apart and their sum is 10 cm.

To Find :

• Its area.

Solution :

We know that,

`{ \qquad \: \pmb{ (1)/(2) * (sum \: of \: the \: parallel \: sides) * height} = \pmb{Area_((trapezium))}}`

Now, Substituting the given values in the formula :

`\qquad { \dashrightarrow \: { \sf{ (1)/(2) * 10\: * 2 \: = {Area_((trapezium))}}}}`

`\qquad { \dashrightarrow \: { \sf{ (1)/(2) * 20 = {Area_((trapezium))}}}}`

`\qquad { \dashrightarrow \: { \sf{ (20)/(2) = {Area_((trapezium))}}}}`

`\qquad { \dashrightarrow \: { \sf{ 10 = {Area_((trapezium))}}}}`

⠀

Hence,

• The area of the trapezium = 10 cm² .

Answer 2

`{10cm}^(2)`

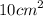
Explanation:

Given,

Sum of parallel sides of the trapezium = 10 cm

Distance between or Height of the trapezium = 2 cm

As we know,

Area of a trapezium

`= (1)/(2) * (sum \: of \: parallel \: sides) * height`

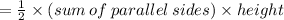
Therefore,

Area of the given trapezium will be,

`= (1)/(2) * 10 \: cm * 2 \: cm`

• (On Simplification )

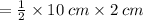
= 1 × 10 cm × 1 cm

• (On multiplying)

`= 10 {cm}^(2)`

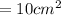
Hence,

The Area of the trapezium is 10 sq.cm (Ans)