Question

Math tangent of a circle Also sorry my sister got a hold of it
ASAP!!!

Answer 1

Imagine we drew a line from the centre (2,0) to the point (0,4).

Essentially the line we drew from the centre to that point on the circle is perpendicular to the tangent line slope-wise.

The slope of that line from the centre to the point is -2 based on the slope's equation or formula.

slope =
`(y2-y1)/(x2-x1)=(4-0)/(0-2) =-2`

Therefore the tangent equation's slope is 1/2 since any perpendicular line's slope is the negative reciprocal of the original line's slope.

By using the point-slope form, we must have the point on the tangent plane (0,4) and the slope we found which is 1/2.

`y - 4 = (1)/(2)(x-0)\\ y= (1)/(2)x + 4`

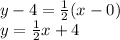
Hope that helps!