To solve
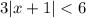 , isolate the absolute value, create two cases, and combine solutions. The correct answer is
, isolate the absolute value, create two cases, and combine solutions. The correct answer is
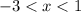 , and the appropriate choice is D.
, and the appropriate choice is D.
Let's solve the inequality step by step:
![\[3|x + 1| < 6\]](https://img.qammunity.org/2023/formulas/mathematics/college/ggywe91n2qlipu0vxz5mzwokupzzlqj1b8.png)
1. Isolate the Absolute Value:
![\[ |x + 1| < 2 \]](https://img.qammunity.org/2023/formulas/mathematics/college/92q797dv840gp5s5rpnmcmjwrpjh4jpk2q.png)
2. Create Two Cases:
- Case one:
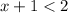
![\[ x < 1 \]](https://img.qammunity.org/2023/formulas/mathematics/college/wh6upl7r7qfp2316mhf3jtahbzu7xbvkld.png)
- Case two:
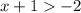
![\[ x > -3 \]](https://img.qammunity.org/2023/formulas/mathematics/college/g83v9ldr1x7zo173lq38dp3u8ogz999x82.png)
3. Combine the Solutions:
- Combine the solutions from both cases:
![\[ -3 < x < 1 \]](https://img.qammunity.org/2023/formulas/mathematics/college/ngz5x16k26hlxd98l22guankxk7v0noojq.png)
Now, let's look at the choices:
A.
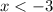 or
or
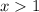 - Incorrect
- Incorrect
B.
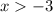 and
and
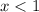 - Incorrect
- Incorrect
C.
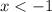 or
or
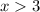 - Incorrect
- Incorrect
D.
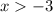 and
and
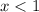 - Correct (it marks the interval from -
- Correct (it marks the interval from -
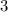 to
to
 )
)
So, the correct solution is
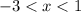 , and D marks the interval from -
, and D marks the interval from -
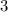 to
to
 , so D is the correct choice.
, so D is the correct choice.