Answer:
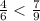 ;
;
 ;
;
 ;
;
 ;
;
 ;
;
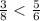 ;
;
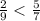 ;
;
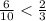 ;
;
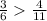 ;
;
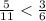
Explanation:
For each comparsion, simplify, find the common denominator, then compare.
(a)
Simplify

Then between 3 and 9, the lowest common denominator would be 9.
This gives:
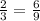 and
and
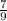 stays the same.
stays the same.
Therefore
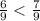 and
and
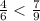
(b)
Simplify

Then between 5 and 9, the lowest common denominator would be 45.
This gives:
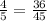 and
and
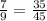
Therefore
 and
and

(c)
Simplify
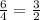
Then between 2 and 5, the lowest common denominator would be 10.
This gives:
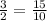 and
and
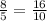
Therefore
 and
and

(d)
Simplify
 and
and
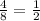
Then between 2 and 6, the lowest common denominator would be 6.
This gives:
 stays the same and
stays the same and
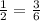
Therefore
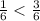 and
and

It's a similar process for the rest and you can always verify your answer with a calculator. If you need more help with the rest just say so! Hope this helps.
Also, when in doubt of finding a common denominator just multiply the two denominators. For example in (b) the lowest common denominator between 5 and 9 = 5*9=45.