Answer:
Our problem is
 , but as we can see, we are unable to factor. We have to use the quadratic equation to solve.
, but as we can see, we are unable to factor. We have to use the quadratic equation to solve.

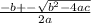
Positive Quadratic Formula:
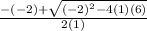
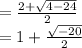
Negative Quadratic Formula:

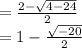
Since both of our answers are the square root of a negative number, we know that the quadratic equation has no real solution.
*We could have also used the Discriminant Test to determine whether the quadratic equation has real roots or not. However, for our means, the quadratic equation seems enough.