Answer:
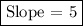
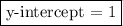
Explanation:
To find the slope of the line, we must pick any two points on the line. Using those two points, we will calculate the Rise and the Run. Then, we will use the slope formula (Rise/Run) to find the slope. The y-intercept is the intersection of the point on the y-axis.

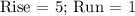
≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡
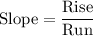
➡
![\bold{Slope = (5)/(1) = \underline{5}} \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ [\text{Rise = 5; Run = 1}]](https://img.qammunity.org/2023/formulas/mathematics/high-school/2jljrg875sueiznzl373ax5tqp3w4ptrag.png)

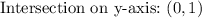
➡
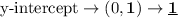
≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡≡