Hi there!
We can use the following equation to solve for equivalent resistance:
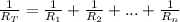
We can plug in the givens and solve.
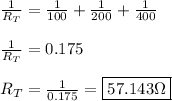
The resistor that would draw the most current is the 100 Ohm resistor because current chooses the path of LEAST RESISTANCE. This can also be proved mathematically with the following:
For resistors in parallel, the POTENTIAL DIFFERENCE (VOLTAGE) is the same.
Since I = V/R, a smaller 'R' means a larger 'I'. Thus, the smallest resistor would have the greatest current through it.