Question

Can someone please help me in one of these!?

Answer 1

9) y = -200x + 1200

11) slope = -2

15) $2,450

16) 9 days

Explanation:

9) Given the two points from the graph:

Let (x₁, y₁) = (0, 1200)

(x₂, y₂) = (1, 1000)

Substitute these values into the following slope formula:

m = (y₂ - y₁)/(x₂ - x₁)

m = (1000 - 1200)/(1 - 0)

m = -200/1

m = -200

The slope of the line is -200.

Next, we need to determine the y-intercept, which is the point on the graph where it crosses the y-axis. Upon observing the graph, it shows that the line crosses at point (0, 1200). The y-coordinate of this ordered pair is the value of the y-intercept, b = 1200.

Therefore, the linear equation in slope-intercept form is y = -200x + 1200.

11) Given the points, (5, -18) and (-4, 0):

Let (x₁, y₁) =(5, -18)

(x₂, y₂) = (-4, 0)

Substitute these values into the following slope formula:

m = (y₂ - y₁)/(x₂ - x₁)

`m = (0 - (-18))/(-4 - 5) = (0 + 18)/(-9) = -2`

Therefore, the slope of the line is -2.

15) Solve:

Given the fixed fee of $200, and the $150 per hour after the initial meeting:

We can represent these in slope-intercept form:

y = 150x + 200

y = total cost for the Attorney's services

x = number of hours worked.

The y-intercept in this given problem is $200, which represents the flat fee charged for the initial meeting. While the slope in this equation is $150, which is the hourly rate that an Attorney charges his clients after the initial meeting.

If an Attorney works for 15 hours, then:

Let x = 15, and substitute its value into the equation to find the total cost:

y = 150x + 200

y = 150(15) + 200

y = 2,250 + 200

y = 2,450

Therefore, a client will pay a total of $2,450 for an Attorney's 15 hours of work.

16) Solve:

Given the water level on a Lake of 165 inches after a rainstorm, and the water level's receding rate of 3 inches per day:

We can establish the following linear equation to model this given problem:

L = -3d + 165

Where:

L = represents the water level of the Lake

d = number of days that the water level recedes

In order to find the number of days it will take before the water level recedes to 84 inches:

Substitute the value of L = 84 into the equation:

L = -3d + 165

84 = -3d + 165

Subtract 165 from both sides:

84 - 165 = 3d + 165 - 165

-27 = -3d

Divide both sides by -3:

`(-27)/(-3) = (-3d)/(-3)`

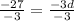
9 = d

Therefore, it will take 9 days for the water level to be at 84 inches.