Question

Over the interval [0,2pi), what are the solutions to cos(2x) = cos(x)? check all that apply​

Answer 1

`\cos(2x) = \cos(x)`

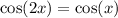
Solution (1)

`2x = x + 2k\pi`

`2x - x = 2k\pi`

`x = 2k\pi`

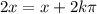
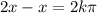
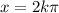
for k=0 / for k=1 / for k=-1

x=0 / x=2π / x=-2π

acc / acc / rej

solution (2)

`2x = - x + 2k\pi`

`2x + x = 2k\pi`

`3x = 2k\pi`

`x = (2k\pi)/(3)`

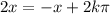
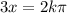
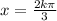
for k=0 / for k=1 / for k=-1

x=0 / x=2π/3 / x=-2π/3

acc / acc / rej

Note that i'm trying values of K which make the answer belong to our interval;

So our solution which i will represent as a set is;

S € {0,2π/3,2π}