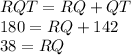Question

Use the image to match the arc/angle measure.

(there is one more option that i wasn’t able to get in the photo it is 67°

Answer 1

The following measurements are:

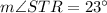`m\angle{STR}=23^\circ` (Option #4)

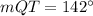`m{QT}=142^\circ` (Option #7)

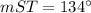`mST=134^\circ` (Option #5)

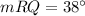`mRQ=38^\circ` (Option #2)

Explanation:

To begin, we can find the measure of
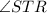`\angle{STR}` by applying the inscribed angle theorem: an angle θ inscribed in a circle is half of the central angle 2θ that subtends the same arc on the circle.

Since the intercepted arc (RS) is 46 degrees, we have:

`46=2\theta\\23=\theta`

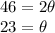
Next, we can find the measure of arc QT using the same theorem. So,

`QT=2(71)\\QT=142`

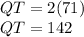
Notice that the chord RT is actually a diameter. From the theorem about the inscribed angle including a diameter, we know that the intercepted arc will have a measure of
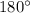`180^\circ`. Since the arc ST is part of the arc RST, and we know RS is
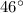`46^\circ`, we can set up and solve this equation:

`RST = RS + ST\\180 = 46 + ST\\134 = ST`

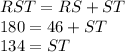
We can use the same idea to find RQ. We know that RQT is
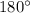`180^\circ` and QT is
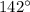`142^\circ`, so:

`RQT = RQ + QT\\180 = RQ + 142\\38 = RQ`