A ratio is a mathematical comparison of two numbers, based on division. For example, suppose you bring 2 scarves and 3 caps with you on a ski vacation. Here are a few ways to express the ratio of scarves to caps
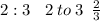
The simplest way to work with a ratio is to turn it into a fraction. Be sure to keep the order the same: The first number goes on top of the fraction, and the second number goes on the bottom.
In practice, a ratio is most useful when used to set up a proportion — that is, an equation involving two ratios. Typically, a proportion looks like a word equation, as follows:
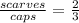
For example, suppose you know that both you and your friend Andrew brought the same proportion of scarves to caps. If you also know that Andrew brought 8 scarves, you can use this proportion to find out how many caps he brought. Just increase the terms of the fraction
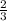
so that the numerator becomes 8. Do this in two steps:
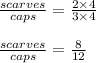
As you can see, the ratio 8:12 is equivalent to the ratio 2:3 because the fractions
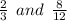
are equal. Therefore, Andrew brought 12 caps
hope it helps