Answer:
y = -⅓x + 3
Explanation:
By definition, parallel lines have the same slope.
Given the linear equation, 3y + x = 6, and the point, (3, 2):
Transform the given equation into its slope-intercept form, y = mx +b, where m = slope, and b = y-intercept.
3y + x = 6
Subtract x from both sides:
3y + x - x = - x + 6
3y = -x + 6
Divide both sides by 3 to isolate y:
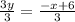
y = - ⅓x + 2 (slope, m = - ⅓, and y-intercept, b = 2).
Since the slope of the given line is - ⅓, then it means that the other line must also have the same slope of - ⅓. All we need to do at this point is to determine the y-intercept of the other line. Using the slope, m = -⅓, and the given point, (3, 2), substitute their values into the slope-intercept form:
y = mx + b
2 = -⅓(3) + b
2 = -1 + b
Add 1 to both sides to isolate b:
2 + 1 = -1 + 1 + b
3 = 0 + b
3 = b
Therefore, the equation of the other line that is parallel to 3y + x = 6 is:
y = -⅓x + 3.