Answer:

Step-by-step explanation:
We are asked to find the force applied to the volleyball. According to Newton's Second Law of Motion, force is the product of mass and acceleration.
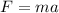
We are given the mass, but we must calculate the acceleration.
1. Acceleration
Acceleration is the rate of change of velocity with respect to change. It is the change in velocity over the time.
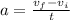
The volleyball starts at rest or 0 meters per second and reaches a velocity of 22 meters per second in 0.2 seconds.
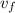 = 22 m/s
= 22 m/s
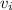 = 0 m/s
= 0 m/s - t= 0.2 s
Substitute the values into the formula.
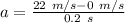
Solve the numerator.
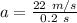
Divide.
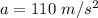
2. Force
Now we know the mass and acceleration, so we can calculate the force.
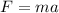
The mass is 0.5 kilograms and the acceleration is 110 meters per second squared.
Substitute the values into the formula.
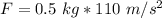
Multiply.
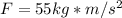
1 kilogram meter per second squared is equal to 1 Newton, so our answer of 55 kg*m/s² is equal to 55 Newtons.
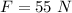
55 Newtons of force are applied to the volleyball.