Question

Write an equation in slope-intercept form of the line shown (1,-9) , (-3,-9)

Answer 1

• 
  `\boxed{\sf{y=-9}}`

Explanation:

The slope-intercept form is the only way to solve this problem.

Slope-intercept form:

`\rightarrow \sf{y=mx+b}`

• The m represents the slope.
• The b represents the y-intercept.

Using the slope is another way to solve.

(1,-9) and (-3,-9)

Use a slope formula.

Slope:

`\sf{(y_2-y_1)/(x_2-x_1)=(rise)/(run) }`

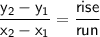
y2= (-9)

y1= (-9)

x2= (-3)

x1= (1)

Rewrite the problem.

`\sf{((-9)-(-9))/((-3)-1)=(0)/(-4)=\boxed{\sf{0} }`

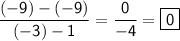
• Therefore, the slope is 0.

We're not done yet.

y-intercept= (-9).

The m represents is 0.

The b represents is (-9).

• So, the correct answer is y=-9.

I hope this helps you! Let me know if my answer is wrong or not.

Answer 2

y = -9

Explanation:

y2 - y1 / x2 - x1

-9 - (-9) / -3 - 1

0 / -4

y = 0 + b

-9 = b