Answer:
Explanation:

Consider
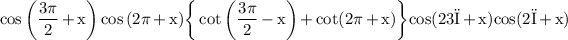
We Know,
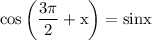
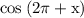
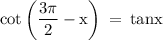

So, on substituting all these values, we get
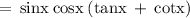
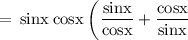
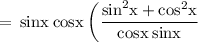
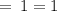
Hence,
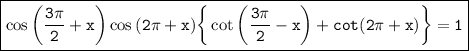
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
ADDITIONAL INFORMATION :-
Sign of Trigonometric ratios in Quadrants
- sin (90°-θ) = cos θ
- cos (90°-θ) = sin θ
- tan (90°-θ) = cot θ
- csc (90°-θ) = sec θ
- sec (90°-θ) = csc θ
- cot (90°-θ) = tan θ
- sin (90°+θ) = cos θ
- cos (90°+θ) = -sin θ
- tan (90°+θ) = -cot θ
- csc (90°+θ) = sec θ
- sec (90°+θ) = -csc θ
- cot (90°+θ) = -tan θ
- sin (180°-θ) = sin θ
- cos (180°-θ) = -cos θ
- tan (180°-θ) = -tan θ
- csc (180°-θ) = csc θ
- sec (180°-θ) = -sec θ
- cot (180°-θ) = -cot θ
- sin (180°+θ) = -sin θ
- cos (180°+θ) = -cos θ
- tan (180°+θ) = tan θ
- csc (180°+θ) = -csc θ
- sec (180°+θ) = -sec θ
- cot (180°+θ) = cot θ
- sin (270°-θ) = -cos θ
- cos (270°-θ) = -sin θ
- tan (270°-θ) = cot θ
- csc (270°-θ) = -sec θ
- sec (270°-θ) = -csc θ
- cot (270°-θ) = tan θ
- sin (270°+θ) = -cos θ
- cos (270°+θ) = sin θ
- tan (270°+θ) = -cot θ
- csc (270°+θ) = -sec θ
- sec (270°+θ) = cos θ
- cot (270°+θ) = -tan θ