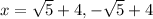Answer:
Explanation:
Take the square root of each side of the equation to set up the solution for x
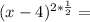 ±
±

Remove the perfect root factor x - 4 under the radical to solve for x.
x - 4 = ±

The complete solution is the result of both the positive and negative portions of the solution.
First, use the positive value of the ± to find the first solution.
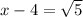
Add 4 to both sides of the equation.
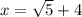
Next, use the negative value of the ± to find the second solution.
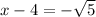
Add 4 to both sides of the equation.
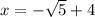
The complete solution is the result of both the positive and negative portions of the solution.