Question

The slope, y-intercept, and write the equation for the line in slope-intercept

Slope
y-intercept
(-5,7)

(-6,-4)

Answer 1

slope is 11

Explanation:

so y = 11x + 62

I used the point slope form to get the y intercept

Answer 2

Hello !

Answer:

`\boxed{ \begin{gathered} \sf \star\ \ y=11x+62 \\ \sf\star\ \ Slope : 11\\\sf \star\ \ y-intercept: 62 \end{gathered}}`

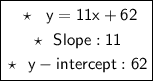
Explanation:

The line pass through the two following points :

• 
  `\sf(-5,7)`
• 
  `\sf (-6,-4)`

The slope-intercept form of a line is of the form
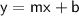`\sf y=mx+b` where m is the slope and b is the y-intercept.

We're looking for the two coefficients m and b.

Let's replace x and y with their values :

`\begin{cases}\sf 7=-5m+b \\\sf -4=-6m+b\end{cases}`

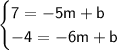
We get a system of two equations to solve.

Let's subtract the second line from the first one and solve for m :
`\sf 7-(-4)=-5m-(-6m)+b-b\\\iff \sf 7+4=-5m+6m\\\iff \boxed{\sf m=11}`

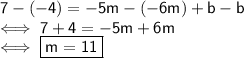
Let's substitute 11 for m in the first equation :

`\sf 7=-5*11+b\\\iff \sf 7=-55+b\\\iff \boxed{\sf b=62}`

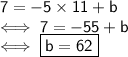
The slope-intercept form of the equation is
`\sf y=11x+62`.

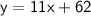The slope is 11.

The y-intercept is 62.

Have a nice day ;)