Question

A 400 g ball swings in a vertical cirde at the end of

a 15-m-long string. When the ball is at the bottom
of the cirde the tension in the string is 10 N.
What is the speed of the ball at that point.

Answer 1

15.10m/s

Step-by-step explanation:

The mass of the ball(m)=400g = 0.4kg

The radius of the string is(r)=15m

The tension in the string is(T)=10N

The acceleration due to gravity =
`9.8m/s^(2)`

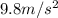
The tension in the string when the body is at the bottom is given by

`T=(mv^(2) )/(r)+mg`

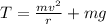
To find the speed of the ball, we make v the subject of the formula

Therefore,
`v=\sqrt(r(T-mg)/(m)`

`v= \sqrt(15(10-0.4*9.8))/(0.4)`

`v=\sqrt(91.2)/(0.4) \\`

`v=\sqrt228 = 15.10 m/s`

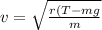
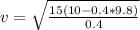
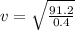
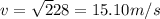
The speed of the ball = 15.10m/s