Question

This diagram shows a park's walking path in the shaded area.

The outside circumference of the walking path is 50.24 meters and the inside circumference of the walking path is 25.12 meters.

What is the area of this walking path?

Use 3.14 for π.

Enter your answer as a decimal in the box.
Point theif = ban

Answer 1

150.72 m²

Explanation:

To find the shaded area, calculate the area of the smaller (white) circle and subtract it from the area of the larger circle.

-------------------------------------------------------------------------------------------

Formulae

• Area of a circle =
  `\pi r^2`
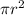
• Circumference of a circle =
  `2\pi r`

(where
`r` is the radius of the circle)

-------------------------------------------------------------------------------------------

Area of large circle

Given:

• circumference = 50.24 m
• 
  `\pi` = 3.14

⇒ 50.24 = 2 × 3.14 × r

⇒ r = 8 m

Therefore:

⇒ Area = 3.14 × 8²

= 200.96 m²

-------------------------------------------------------------------------------------------

Area of small circle

Given:

• circumference = 25.12 m
• 
  `\pi` = 3.14

⇒ 25.12 = 2 × 3.14 × r

⇒ r = 4 m

Therefore:

⇒ Area = 3.14 × 4²

= 50.24 m²

-------------------------------------------------------------------------------------------

Area of shaded path

area = area of large circle - area of small circle

= 200.96 - 50.24

= 150.72 m²

-------------------------------------------------------------------------------------------