Answer:
The area of the smaller triangle is 80m²
Explanation:
Find the scale factor to get the dimensions of the smaller triangle from the larger triangle using the given information:

When scaling the area of similar shapes, we will square the scale factor for the lengths. We do this because area is a 2-dimensional measurement.
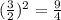
Let x represent the area of the smaller triangle. So, we have:
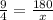
Cross multiply:
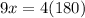
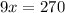
Divide by 9:


Therefore, the area of the smaller triangle is
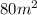 .
.