Answer:
Length = 8 meters
Width = 6 meters
Explanation:
Given the following information:
Perimeter of a rectangular vegetable patch: 2(L + W) = 28 meters
Area = L × W = 48 m²
We can solve for the dimensions by using both equations.
First, let's use the formula for the perimeter of the rectangular vegetable patch, and isolate one of the given variables:
2(L + W) = 28
Divide both sides by 2:
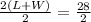
L + W = 14
Subtract W from both sides to isolate L:
L + W - W = 14 - W
L = 14 - W
Next, we'll take the formula for the area, and substitute the value for the L from our previous step:
A = 48 = L × W
48 = W × (14 - W)
Distribute W into the parenthesis:
48 = 14W - W²
Add W² and subtract 14W to both sides:
W² - 14W + 48 = 14W - 14W - W² + W²
W² - 14W + 48 = 0 ⇒ This represents a quadratic equation in standard form. We can use the coefficient and constant values to solve for its roots.
a = 1, b = -14, and c = 48
Substitute these values into the quadratic equation:


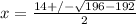
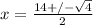
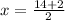 ,
,
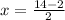
x = 8, x = 6
Now, we can substitute these values into the formulas for the perimeter and area to find the true dimensions of the rectangular vegetable patch.
Perimeter: 2(L + W) = 2(8 + 6) = 28 meters
Area = L × W = 8 × 6 = 48 m²
Therefore, the dimensions of the rectangular vegetable patch are:
Length = 8 meters
Width = 6 meters
Dimensions: 8 × 6 meters.