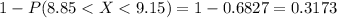Answer:
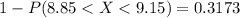
Explanation:
We need to first calculate the probability that a unit between the weight of 8.85 and 9.15 ounces is NOT a defect, then subtract it from 1.
It just so happens that 8.85 and 9.15 are both 1 standard deviation from the mean of 9, so by the Empirical Rule,
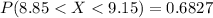 .
.
Thus, the probability of a unit being a defect is