
First equation is
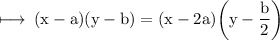
can be further simplified to
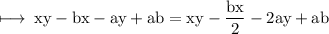

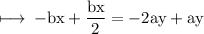
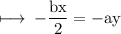
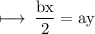
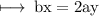
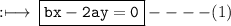
Second equation is
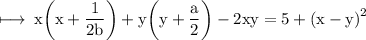
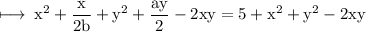
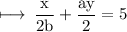
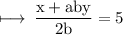
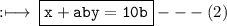
So, we have two equations in simplest form as

and

Now, Consider
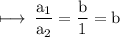

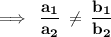
This implies, System of equations is consistent having unique solution.
So, The student is correct as lines are intersecting.
So, option (b) is correct.