Answer:

Explanation:
We are given the function:
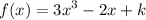
Where (x + 2) is a factor of f. And we want to determine the vale of k.
Recall that from the Factor Theorem, if (x - a) is a factor of a polynomial P(x), then P(a) must equal 0.
We can rewrite our factor as (x - (-2)). Hence, a = -2. Our polynomial is f. Since (x + 2) is a factor, then from the Factor Theorem, f(-2) must be 0.
Using this information, we can now determine k:

In conclusion, the value of k is 20.