Question

Lucy has read 500 pages of her summer reading assesment, and Angie has read 460 pages. Lucy has reads 10 per week for the remainder of the summer, and Angie reads 20 pages per week for the remainder of the summer. Write and solve an equation to show how many weeks it will take before the girls have read the same amount of pages.​

Answer 1

It would take four more weeks before the two read the same number of pages.

Explanation:

Let
`x` denote the number of extra weeks required before the two read the same number of pages.

Express the number of pages each would read between now and
`x` weeks into the future:

Lucy reads
`10` pages per week. Thus, she would have read
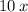`10\, x` additional pages after
`x` more weeks.

Angie reads
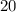`20` pages per week. She would've read
`20\, x` additional pages after
`x` more weeks.

It is also given that Lucy has already read
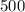`500` pages. After reading
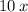`10\, x` pages in the upcoming
`x` weeks, Lucy would have read a total of
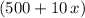`(500 + 10\, x)` pages.

Likewise, after reading
`20\, x` pages in the upcoming
`x` weeks, Angie would have read a total of
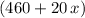`(460 + 20\, x)` pages.

It is assumed that the two would have read the same number of pages after these
`x` more weeks. In other words:

`500 + 10\, x = 460 + 20\, x`.

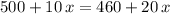Solve this equation for
`x`:

`x = 4`.

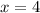In other words, Lucy and Angie would have read the same number of pages after four more weeks.