Question

I am completely braindead on how to calculate the time first.

A flowerpot falls from a window sill 31.3m from a sidewalk. What is the velocity of the flowerpot when it hits the ground? (hint: Use d=1/2at^2 to find "t" first, then find velocity using v=at. Remember, ay= -9.8 m/s2).

Answer 1

24.8 m/s

Step-by-step explanation:

You don't have to solve for time first. You can use the equation below to solve for the velocity before impact:

`v_y^2 = v_(0y)^2 + 2a_yy`

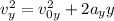
We know that
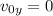`v_(0y) = 0` so the equation above becomes

`v_y^2 = 2a_yy \Rightarrow v_y = √(-2a_yy)`

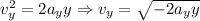
Plugging in the numbers, we get

`v_y = \sqrt{-2(-9.8\:\text{m/s}^2)(31.3\:\text{m})}`

`\:\:\:\:\:=24.8\:\text{m/s}`

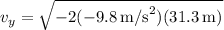
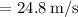
METHOD 2:

If you insist on using that equation for d, we can do that too. So solving for t from the equation for d. we get

`t = \sqrt{(2d)/(g)} = \sqrt{\frac{2(31.3\:\text{m})}{9.8\:\text{m/s}^2}}`

`\:\:\:\:= 2.53\:\text{s}`

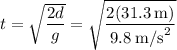
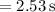
So using the equation
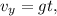`v_y = gt,` we get

`v_y = (9.8\:\text{m/s}^2)(2.53\:\text{s}) = 24.8\:\text{m/s}`

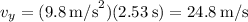
So you get the same result regardless of the method you use.