Question

The points (6, 9) and (5, 8) fall on a particular line. What is its equation in slope-intercept form?

Answer 1

y=x+3

Explanation:

Start with the slope equation
`(y_(2) -y_(1) )/(x_(2) -x_(1) )`

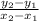
Since the first point is actually further along the x-axis that x-value becomes
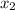`x_(2)` and its corresponding y-value is
`y_(2)`

`(9-8)/(6-5)`=
`(1)/(1)`

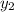
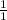
The slope for the equation is just one so now find the y-intercept by rearranging the slope-intercept equation.

y=mx+b

y-mx=b

Now Substitute either point in to find b

9-1(6)=b

9-6=b

b=3

Your y-intercept is 3 so now we can write the slope-intercept equation for the two points:

y=x+3