Explanation:
Let us assume the ratio's as 4x, 5x and 6x.
A.T.Q
6x+4x=5x+55
→ 10x = 5x + 55
→ 10x - 5x = 55
→ 5x = 55
→ x =
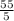
x = 11
Therefore,
First no. = 4x = 4 × 11 = 44
Second no. = 5x = 5 × 11 = 55
Third no. = 6x = 6 × 11 = 66
Hence the three numbers are 44,55 and 66.